IRAS Explanatory Supplement
VII. Analysis of Processing
D. Photometric Accuracy
D.2 Relative Photometric Accuracy
Table of Contents | Index | Previous Section | Next Section
A global view of the quoted relative photometric errors of the catalog is shown in Table VII.D.2 including all sources of moderate or high quality flux densities (Section V.H.5). As with the analysis of the positional accuracy, the analysis of the photometric accuracy was done separately for bright sources, with flux densities greater than 1.2, 1.5, 1.9 and 4 Jy at 12, 25, 60 and 100 µm,and faint sources, with flux densities below these limits. In addition, the effects of excluding sources with a quoted probability of true variability greater than 50% at 12 and 25 µm (Section V.H.5) and sources in high source density regions (see Section V.H.6.a) are shown. In all cases, discrepant fluxes (i.e. sources with HCON-to-HCON flux ratios showing reduced
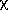2 greater
than 9; see Section V.H.5) were excluded from the
samples.
| wavelength (µm) | Number Sources | Bright Sources Mean1 Unc. | Mean No. HCON | Number Sources | Faint Sources Mean Unc. | Mean No. HCON |
|---|---|---|---|---|---|---|
| 12 | 61,653 | 0.09 | 3.2 | 97,122 | 0.10 | 2.9 |
| 25 | 32,916 | 0.ll | 3.2 | 67,652 | 0.13 | 3.2 |
| 60 | 23,335 | 0.14 | 3.1 | 47,342 | 0.11 | 2.8 |
| 100 | 43,562 | 0.15 | 3.0 | 43,562 | 0.13 | 2.7 |
| 12 | 39,029 | 0.07 | 3.2 | 86,962 | 0.10 | 2.9 |
| 25 | 17,320 | 0.09 | 3.2 | 56,682 | 0.13 | 3.2 |
| 60 | 9,437 | 0.11 | 3.0 | 43,012 | 0.11 | 2.8 |
| 100 | 7,080 | 0.11 | 2.8 | 34,593 | 0.12 | 2.6 |
| 12 | 30,228 | 0.06 | 3.1 | 82,869 | 0.10 | 2.9 |
| 25 | 11,586 | 0.07 | 3.1 | 48,609 | 0.125 | 3.2 |
| 60 | 8,055 | 0.11 | 3.0 | 4l,056 | 0.11 | 2.8 |
| 100 | 6,809 | 0.11 | 2.7 | 34,358 | 0.12 | 2.6 |
|
1 Mean unc. is the quoted fractional uncertainty 2 Sources deemed variable at 12 and 25 µm were not used at 60 and 100 µm | ||||||
Tests of the photometric accuracy were made by comparing the flux densities obtained for a given source on all possible pairs of HCONs. The ratio of two hours-confirmed flux densities belonging to a weeks-confirmed source is a random variable whose variance should be the sum of the variances in the two individual hours-confirmed flux densities.
D.2.a Relative Photometric Accuracy of HCONs
 |
|
Figure VII.D.4 Histograms of ratios of pairs of HCON Measurements
for faint sources in the four wavelength bands. larger largest |
Figures VII.D.4 and VII.D.5 show histograms of the natural log of ratios of HCON flux densities for bright and faint weeks-confirmed sources. The samples were the bottom set described in Table VII.D.2.The flux density chosen to be in the numerator of the ratio is the one with the higher flux status (see Table V.D.5) or the later time of observation in cases of equal flux status.
The distributions in Fig. VII.D.4 and VII.D.5 were fitted to
Gaussian distributions using only data above 25% of the peak value of the
distribution. This choice fits the observations in the central region; the
excess in the wings becomes quite visible. The significant non Gaussian
component is thought to come primarily from the effects of particle radiation,
and is especially obvious for the brighter sources. In addition to particle
radiation effects, there may also be some contribution due to intrinsic
variability(which was significantly reduced in the samples discussed here),
cross-scan variations in detector sensitivity particularly at 60 and 100
µm and the systematic selection of brighter fluxes at points in the
processing where the measurements do not qualify for refinement
(Section V.D). All of these effects are
probably masked in fainter sources by their larger Gaussian noise.
 |
|
Figure VII.D.5 Histograms of ratios of pairs of HCON measurements
for bright sources in the four wavelength bands. larger largest |
The exact nature of the particle radiation effects on the photometry is not well understood, but it is clear that some subtle phenomena are involved. Large spikes were suppressed by the deglitcher circuits, and were most likely to inhibit the detection of a real point source, although a detection on with a large photometric estimation error could result. Small spikes may easily have been lost in the other noise process. Intermediate spikes tended to cause flux overestimation in cases which have been examined, but whether this led to setting the discrepant-flux flag depended on the actual brightness of the source relative to the spike as well as the uncertainties assigned to the fluxes.
Table VII.D.3 lists the widths (1-
) of the Gaussian fits to the
ln(fv(2)/fv(1)) distributions shown in
Fig. VII.D.4 andVII.D.5. If the
variance of these distributions is twice the mean variance of a single HCON
and if the catalog flux densities are generated from an average of three
HCONs, the expected mean catalog relative photometric uncertainties would be
0.03, 0.03, 0.04 and 0.05 for bright sources in the 12, 25, 60 and 100
µm bands, respectively. This estimate of catalog flux density
uncertainties is based on the intrinsic uncertainty of HCON pairs and is
substantially smaller than the mean uncertainties listed in the catalog
(Table VII.D.2). For the 12, 60 and 100 µm bands
the differences can be attributed to effects of the non-Gaussian wings,
although in the 25 µm band the non-Gaussian wings are quite small.
D.2.b The Quoted Flux Density Uncertainties
The uncertainties in the quoted flux densities are based on a statistical evaluation of the consistency of HCON ratios. As discussed below, the IRAS survey produced highly repeatable flux densities and therefore small quoted uncertainties. Systematic effects on the photometry, such as flux-dependent nonlinearities, are discussed in Chapters IV and VI and almost certainly dominate the true photometric uncertainties, especially for the brighter sources and longer wavelengths.
| wavelength (µm) | Bright Sources | Faint Sources | ||
|---|---|---|---|---|
| Number | Gaussian1 | Number | Gaussian |
|
| 12 | 107,932 | 0.074 | 245,465 | 0.15 |
| 25 | 44,551 | 0.072 | 145,069 | 0.15 |
| 60 | 25,867 | 0.096 | 101,274 | 0.15 |
| 100 | 17,234 | 0.13 | 72,003 | 0.18 |
| 12 | 107,932 | 0.65 | 245,465 | 0.92 |
| 25 | 44,551 | 0.51 | 145,069 | 0.71 |
| 60 | 25,867 | 0.44 | 101,274 | 0.77 |
| 100 | 17,234 | 0.60 | 72,003 | 0.87 |
|
1 The Gaussian is derived from the fit to the upper
75% HCON pairs in Figs. VII.D.4, D.5, D.7,
D.8 2 The HCON uncertainty is the square root of the sum of the squares of the individual HCON uncertainties. | ||||
The repeatability of the quoted flux densities was assessed by a comparison of the flux ratio of two hours-confirmed flux densities belonging to a weeks-confirmed source with the HCON uncertainty, defined as the square root of the sum of variances of the hours-confirmed flux densities. The data of two such hours-confirmed flux densities divided by the square root of the sum of the variances should be a random variable with unit mean and unit variance.
 |
|
Figure VII.D.6 Histograms of ratios pairs of HCON measurements
normalized to the resultant HCON uncertainty for bright 12 µm
sources. A Gaussian fit to all of the data is shown. larger largest |
Figure VII.D.6 shows such a histogram for the entire
HCON sample in the 12 µm band including the Gaussian curve which
best fits all the observations in the histogram. It can be seen that the fit
is somewhat crude. The wings of the distribution do not fall off rapidly
enough to be Gaussian, and so the fit acquires too large a variance. In this
figure, the standard deviation of the Gaussian curve is 1.07. The difference
from unity is the residual error in the assignment of photometric
uncertainties at seconds-confirmation and the error associated with the
Gaussian assumption used in flux refinement at seconds and hours-confirmation.
 |
|
Figure VII.D.7 Histograms of ratios of pairs of HCON measurements
normalized to the resultant HCON uncertainty for bright sources
in the four wavelength bands. A Gaussian fit to the central portion
of the data is shown. larger largest |
Figures VII.D.7 and VII.D.8 show
histograms of the HCON-to-HCON flux density ratios divided by the resultant
HCON uncertainty defined above for the bright and faint sources in the
selected sample. Included on each plot are the Gaussian fits using only data
above 25% of the peak value, while Table VII.D.3 shows the
widths (1-) for the fits. The distributions
typically show Gaussian variances significantly less than unity accompanied
by substantial non-Gaussian wings. Together these two distributions produced
the total photometric dispersion which led to the uncertainties quoted in the
catalog.
 |
|
Figure VII.D.8 Histograms of ratios of pairs of HCON measurements
normalized to the resultant HCON uncertainty for faint sources
in the four wavelength bands. A Gaussian fit to the central portion of the data is shown. larger largest |
The small variances of the fits imply that the quoted flux density
uncertainties have been overestimated, but this may well be an artifact of the
selection criteria used to establish the sample. This suggestion is supported
by the fact that during processing more reasonable
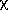2 test results were obtained. In
the 12, 60 and 100 µm bands the small variance may be due to fitting
only the central portion of the distribution and excluding the broad wings.
For the brightest objects, the small variance may be indicative of the true
Gaussian dispersion which is superimposed on the broader distribution in the
tails.
The brightest and faintest 10% of the sources in the samples show the
same effects as discussed above in an enhanced manner. In addition, the
faintest sources show a statistically significant deviation in the mean of
about 2% although the fitting process assumed a mean of zero. This would
slightly inflate the derived standard deviation of the Gaussian fit. The
brightest sources show a small non-zero mean in the opposite direction from
that of the faint sources. These effects are not understood.
Table of Contents | Index | Previous Section | Next Section